Science in Christian Perspective

 

 

The Astrophysics of Worlds in Collision
 ROBERT C. NEWMAN
Biblical School of Theology 
Hatfield, Pennsylvania 19440

From: JASA 25 (December 1973): 146-151.
Robert C. Newman has a Ph.D. from Cornell University in theoretical astrophysics (1967), as well as five years of graduate training in theology.
Introduction

The solar system has been the scene of repeated catastrophes in the millennia preceding the time of Christ, according to Immanuel Velikovsky.1 In his book Worlds in Collision, first published in 1950, the author concentrates on the two most recent alleged upheavals: our near collision with Venus about 1500 BC, and the close approach of Mars in the period 800-600 BC. In addition, Velikovsky gives a few hints that the deluge of Noah involved a similar phenomenon.2 Jupiter also is thought to have figured prominently in some previous eatastroplse.3 Although Velikovsky apparently intended to devote another hook to these earlier events, he seems to have been side-tracked by adverse reaction to Worlds its Collision.4

In any case, Velikovsky believes that Venus was formed by being thrown out of Jupiter during a near collision among the outer planets.5 At first Venus became a comet with an elongated orbit extending from near Jupiter inward past the earth's orbit.6 On several occasions in the midst of the second millennium BC, the comet Venus approached the earth, producing a variety of physical calamities, including destructive meteor shnwers,7 extensive volcanic flows,8 huge tidal waves,9 the tilting of the earth's axis and changes in its speed of rotation,"' as well as years of semi-darkness during which the sun was obscured.11

Velikovsky cleverly ties these phenomena to the events of the Exodus of the Israelites from Egypt and the conquest of Canaan: the ten plagues,12 the crossing of the Red Sea13 and the Jordan River,14 the manna in the wilderness,15 the smoke, fire and sounds at Mount Sinai,16 the cloudy and fiery pillar,17 the fall of Jericho,18 the "hailstones"19 and Joshua's long day20 at Gibeon. Velikovsky also seeks to synchronize these calamities with the fall of Egypt's Middle Kingdom,21 the migrations of several ancient peoples,22 and the origin of a substantial amount of mythology in all parts of the world.23
Having disrupted the earth's motion, destroyed a number of civilizations, created several new religions, and dumped enormous amounts of manna (=nectar = ambrosia) 24 and petroleum25 on the earth, the comet
Venus continued to threaten the earth for centuries, although no further catastrophes occurred, Finally, about 800 B.C., Venus nearly collided with the planet Mars. As a result, the Martian surface was devastated and its orbit was disrupted, while Venus settled into a new orbit where it became a planet and no longer menaced the earth.26
Unfortunately, however, the new orbit of Mars now made it a threat to earth in place of Venus. Although the Martian upheavals were not so violent as the earlier Venerian calamities,27 the red planet still succeeded in turning hack the shadow on the dial of Ahaz,28 wiping out the Assyrian hosts of Sennacherib besieging Jerusalem,29 providing phenomena for the striking catastrophes mentioned by several of the Old Testament prophets,30 changing the length of the month and the year,31 influencing the outcome of the Trojan War,32 and adding a new war god to the pantheon of many pagan religions.33

Velikovsky and Christianity

It should be clear from this sketch of Velikovsky's reconstruction of ancient history that his work is a matter of concern for Christians. Although he gives a certain amount of historical credit to the Old Testament narratives, Velikovsky is no believer in supernatural revelation.34 Instead he seeks to give a purely naturalistic explanation for the biblical events just as he does for the pagan mythology.

Some Christians, however, have been impressed by the fact that Velikovsky repudiates the uniformitarian outlook of science and that he admits the historicity of certain biblical catastrophes. Among several Christian writers who seem to have been influenced by him, one in particular-Donald W. Patten-seems to have attempted a "Christianization" of Velikovsky's general outlook in his work The Biblical Flood and the Ice Epoch.35 As a result, a number of astrophysical problems in work are shared by that of Patten.

Problems of Orbital Mechanics

In an article of this nature, there are severe limitations of space. Therefore we confine this discussion to two major areas of Velikovsky's astrophysics: orbital mechanics and rotational mechanics.

Velikovsky spends several pages discussing the old nebular and tidal theories for the origin of the solar system, finding them both inadequate.36 It is a fact that the problem of the origin of the solar system is a difficult one, hot many of the problems raised by Velikovsky have been solved.37 Indeed, it seems that an excellent fit can be made between Genesis 1 and Hoyle's model for the formation of the solar system.38

Patten's alternative model is to suggest that the planets were randomly captured by our sun from interstellar space.39 While Velikovsky does not explicitly commit himself to such a scheme, he does suggest that the present orbits of the planets are due to near collisions which involved drastic changes in the orbits of Venus, Mars and several of the outer planets .40

But there are two very serious problems of orbital mechanics which must he faced by such random capture or collision views. (1) The planetary orbits all lie very nearly in the same plane, and (2) these orbits are all very nearly circular.
Relative to the orbital plane of the earth, the 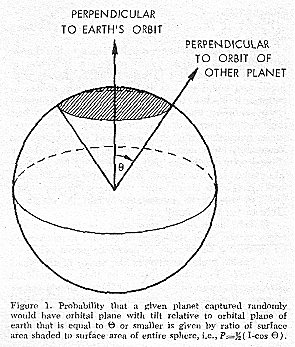orbital planes of the other planets are tilted by the amounts indicated in Table I. If the planets were randomly captured or involved in numerous collisions, we would expect their orbital planes to he randomly oriented, but they are not. To indicate just how unrandom the orientation is, let us calculate the probability that the planets would have as little tilt as they do if they had been captured randomly. The total probability is just the product of the probabilities for each planet separately.


 


The probability for a single planet can be calculated with the help of Figure 1. Drawing a sphere whose equator is the earth's orbit and whose vertical is perpendicular to that orbit, then a planet with orbital plane tilted by an angle & has its perpendicular fall somewhere on the cone shown in Figure 1. Any planet with less tilt has a perpendicular lying within the shaded area. Therefore the probability that a randomly captured planet has a tilt less than or equal to 0 is just the ratio of the shaded area to the area of the whole sphere. Without going through the derivation, this ratio and probability is
P = 1'2' (1 - cos theta)
The result for each planet is given in Table I. The total probability is

                          P tot = (P Merc ) (P Ven ) (P Mars) ... (P Pluto)  which is P tot =6.9 x 10-26.

Since this probability is roughly equal to the chance of finding one marked penny among a collection in which every inhabitant of half a million planets with population equal to ours each has a billion dollars in pennies, it is clear that Patten's proposal lacks merit! Even assuming that only Venus and Mars have had their orbits disrupted (and Velikovsky certainly assumes more than this), the combined probability that Venus and Mars would have orbital planes as closely aligned with earth's as they do is 2.7 x 10 , about one chance in four million. If you knew that someone in Virginia had stolen your wallet, a "random capture" of one of its inhabitants would be about as likely to net the culprit!

In fact, these figures show that any theory for the origin of the solar system must contain a mechanism for producing the planets in nearly co-planar orbits.

A similar problem involves the shape of the planetary orbits. Two objects hound by their gravitational attraction move in ellipses around their common center of mass. For the solar system, this means that each planet moves very nearly in an ellipse about the sun, since the effect of the planets on each other is very small (at least at the present time). The shape of an ellipse is indicated by a quantity called eccentricity, usually represented by the letter e. If e is zero, the ellipse is actually a circle. As e increases from zero toward one, the ellipse becomes more elongated, going to a straight line or parabola as e goes to one. If a planet has been captured randomly or its motion around the sun has been randomly determined by close approaches to other planets, then all values of eccentricity between zero and one should be equally likely.

In fact, the eccentricities of the planets are unusually small, as seen in Table II. The orbits are much more circular than would be expected for random capture or close approaches. This may be seen by calculating the individual and collective probabilities for such small values of e. Assuming that each value of a between zero and one is equally likely, then the probability that e has a value less than or equal to some value x (between zero and one) is just the value x itself.

 

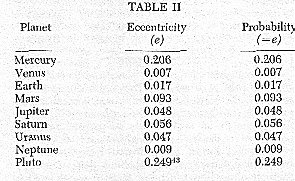
Thus, for instance, Mercury has an eccentricity of 0.206. The probability that a planet captured randomly would have an eccentricity less than or equal to 0.206 is just 0.206. The probability for the whole set of nine planets having as circular orbits as they do is again the product of the individual probabilities. i.e., 6.5 x 10-13, which again is very small. The probability that just Venus, Earth and Mars would be as circular as they are is only 1.1 x 10, again suggesting that the planets have not been randomly captured or greatly disrupted in their orbits.

Although he gives a certain amount of historical credit to the Old Testament narratives, Velikovsky is no believer in supernatural revelation.

In contrast, the comets, which may well have been captured randomly from interstellar space, have much higher eccentricities. The twenty-five short-period comets listed by Motz and Duveeu range in eccentricity from 0.132 to 0.967 with an average just over 0.644 This is not a representative sample of the comets either, because must of the comets known have very long periods and eccentricities very close to one.

For the theories of either Velikovsky or Patten to stand up, they need to find some mechanism which circularizes the orbits of (at least) Venus and Mars. No known physical laws will do the job, and the fact that Halley's comet has been observed to return regularly since 240 B. C.45, indicates that it has suffered little change in eccentricity its the past 2000 years.

Problems of Rotational Mechanics

Now we consider the physical problems involved in stopping the rotation of the earth or drastically tilting its axis of rotation. The physical laws governing the rotation of a rigid object are not as familiar to most people as those controlling the movement of an object from place to place. However, the formulas for rotational motion are really rather similar to those for translational motion except that a number of new quantities are defined. Thus the mass is is replaced by the moment of inertia I, the force F by the torque T, the linear momentum p by the angular momentum L, and the linear velocity v by the angular velocity w. See Table Ill for a comparison of three basic formulas.

We now calculate what torque would be necessary to stop the earth's rotation in a relatively short time. Assuming a constant torque, we can integrate the last equation in Table III to get T=(L/t). The Smithsonian Physical Tables give the mass of the earth as 5.975 X 1027g., its equatorial radius as 6,378 km,46 and its moment of intertia as I= 0.333 mr2,47 from which we calculate I = 8.12 x 1044g cm2. The angular velocity of the earth is 7.3 x 10-5 radians/sec,48 so that we may calculate its angular momentum from the first rotation formula in Table III as L = 5.93 x 1040 g em2,'see.

If the reversal of the shadow of the dial of Ahaz (2 Kings 20) is understood as a reversal of the earth's rotation, then the earth must be brought to a stop in just a few minutes. Joshua's long day requires a stop in a fraction of a day (and a drastic tilt involves about the same change in momentum, and therefore the same torque). Using one full day for the time taken to stop the earth, we find a torque of 6.86 x 1035 dyne cm is necessary.


                                                                      TABLE III    

              Name                  Translation Formula                 Rotation  Formula
Momentum                                p=mv                                     L=Iw

Kinetic Energy                           E=1/2mv2                               E=i/2Iw2

Change in Momentum                F=dp/dt                                  T=dL/dt  


So far, none of these figures make much of an impression unless one is thoroughly familiar with the quantities involved. But now we consider how this torque might he obtained.

Could the torque have been exerted by the gravitational pull of Venus passing very close to the earth? If we took Venus and the earth as rigid spherical objects, the answer is "no," for gravity would have nothing to "grab" to produce a torque. But the close approach of Venus would raise a tidal bulge on the earth, which we can represent by thinking of the earth as composed of two masses, each equal to urn, separated by a small distance 2a. In this ease, the planet Venus, with mass M, at a distance R from the earth (where R is much larger than a) would exert a torque on the earth given by
                             
                                            T= (C M m a2/ 'RI) sin 2 0, 

where C is the universal constant of gravitation and 0 is the angle between Venus and the earth's bulge. See Figure 2 for the geometry of this situation.

Let us choose the optimum value of 45 deg for 0.. For we use the necessary torque calculated above. For simplicity, we use the earth's mass for both in and M. For a, let us choose the value 100 kilometers (about 60 miles), which would represent about as large a bulge as possible without producing large rips in the earth's crust (the moon only produces a tidal bulge in the solid earth of a few inches). Putting all these quantities in the last formula, we solve for R to see how close Venus must come to produce enough gravitational torque to stop the earth's rotation in one day. The result is R = 7000 km, less than 5000 miles! But this is inside the Roche Limit,49 which marks the point at which gravity will pull apart a body approaching the earth. Consequently, it appears that the earth and Venus would disintegrate before they could get close enough together to stop the earth's rotation in one day!

Any theory for the origin of the solar system must contain a mechanism for producing the planets in nearly coplanar orbits. Since magnetism is a stronger force than gravity, Velikovsky seems to he inclined to look in that direction for the necessary torque.50 The earth already has a magnetic field of its own, so if Venus could somehow apply a strong magnetic field to the earth, it might he able to tilt it or stop its rotation. The torque T which can be exerted by a magnetic field B oil an object having a magnetic moment u (sinder optimum conditions) is T =uB.51 Using the required torque 6.86 x 1035 dyne cm, and the earth's



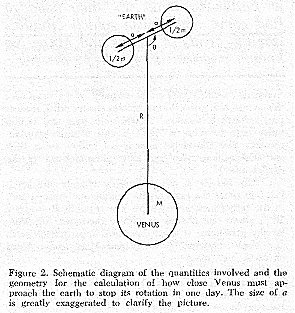
Figure 2. Schematic diagram of the quantities involved and the geometry for the calculation of how close Venus must approach the earth to stop its rotation in one day. The size of e is greatly exaggerated to clarify the picture.

 

magnetic moment u = 8.06 x 1025 egs units,52 the magnetic field required is B = 8.53 x 109 gauss!

For the sake of comparison, the earth's own magnetic field at the surface is about 0.5 gauss; the strong magnetic fields in sunspots are a few thousand gauss; and the largest sustained magnetic fields produced by man are a few hundred thousand gauss. Thus a field of over 8 billion gauss sustained for a day thousands of miles from its source (Venus) is preposterous. Velikovsky seems to sense the force of this problem, and he tentatively suggests an earth-sized mass of iron filings (!) to do the job.53 Just how these all get together to form the planet Venus after the job is done is not specified. In any ease, one shudders to think what sort of remanent magnetism would have been left in our iron deposits if the earth had ever been subjected to such a strong field!

This does not end Velikovsky's difficulties with rotational mechanics, however. It is easy to show that there is only a miniseule probability that Venus would even pass as close to the earth as 6000 miles (in order to influence its rotation) under the conditions Velikovsky specifies. For according to Velikovsky, Venus had an elliptical orbit from about 1500 B.C. to about 650B.C., during which time its orbit presumably extended from about Jupiter's orbit inward to about Venus' present orbit. In such a case, Venus would have an orbital period of at least five years, and therefore it would cross the earth's orbit no more than 340 times.

Assuming that Venus' orbit at that time had a tilt of only 1 deg (smaller than the present tilt of Venus or its "parent" Jupiter), Venus could be as much as 1.7 million miles above or below the earth's orbit when it passed by, of which only the central 12 thousand miles would be close enough to the earth. But Venus must not only come this close to the earth's orbit, the earth must be nearby when it does. Since Venus is moving at least as fast as the earth when it crosses its orbit, the earth moves no more than 12,000 miles while

For the theories of either Velikovsky or Patten to stand up, they need to find some mechanism which circularizes the orbits of Venus and Mars. No known physical laws will do the job.

Venus is within 6,000 miles radially of its orbit. All this means that when Venus crosses earth's orbit, it must pass through a "window" 24,000 miles long and 12,000 miles high to score a "hit"! If this sounds easy, remember that the target area is 3.4 million miles high and 600 million miles long (the circumference of the earth's orbit)!

With 340 shots, the probability of only one hit is just 340 times the ratio of the window or bullseye area to the target area as a whole. The result is P = 4.8 x 10, about one chance in twenty thousand. Yet Velikovsky postulates at least one close approach of earth and Venus, another of Venus and Mars, and a third of earth and Mars!

As in the case of orbital mechanics, there are also some historical evidences against any past permanent changes in the axis or rate of the earth's rotation. The Palermo Stone from Egypt's Old Kingdom (thus before the Venus catastrophe which supposedly destroyed the Middle Kingdom) seems to indicate a 365-day year.54 Unless this evidence can be discounted, Velikovsky would have to postulate compensating changes in the length of both the day and the year to preserve this ratio.

In addition, the pyramids of Cheops and Chephren (also dating from the Old Kingdom) are aligned to present true north within five minutes of arc  (1/12 of a degree). 55 On Velikovsky's assumption that the earth has been tilted, there would be no physical way for the earth to "remember" and return to its previous alignment. Of course, it might he claimed that the earth was previously aligned 90° or 180° away from its present orientation, but even so the probability of such an accurate realignment is P= (1/12°)/90° =9.26x 10-4, about one chance in a thousand!

Conclusions

Considerations of space have precluded a more thorough examination of orbital and rotational problems associated with Velikovsky's (or Patteo's) scheme. It is regretted that most derivations have been left out, but a college-level physics major should be able to reproduce them for himself, and anyone with less training has probably found this paper tedious enough already.

I had intended to devote another section to Venus as a comet, as Velikovsky's thesis has several weak points in this area, but again space forbids. I just observe that Venus' total mass is probably a million times larger than that of any known comet, as is its density,56 and that a comet tail, being extended by the force of the solar wind, could not possibly transport enough material to supply the earth's petroleum even if it could penetrate the earth's magnetosphere and atmosphere (which is unlikely).

In conclusion it appears that Velikovsky must at least postulate the existence of a number of rather specific unknown physical laws if he is to carry out his thesis. While it is very likely that there are still many physical laws unknown to man, it is extremely unlikely that we have missed any forces lurking in the solar system with the strenth and range necessary to produce the effects Velikovsky must have.

My advice to fellow Bible-believers seeking to understand Joshua's long day, the flood, and other spectacular physical phenomena which have occurred during human history is not to quit looking, for very few discoveries are made by people who are not searching for something. But I do suggest that this particular line of investigation-the near collision of planets within the span of human history-is a dead end.

REFERENCES

1Immanuel Velikovsky, Worlds in Collision (New York: Dell-Laurel, 1967), pp. 373-75. Hereafter WIG. The pagination differs in the Doubleday and the larger-format Dell editions,
2WIG, pp. 12, 381. 
3WIG, pp. 182, 381-82.
4Immanuel Velikovsky, Earth in Upheaval (New York: Dell-Laurel, 1968), p. 6.
5WIC, p. 374.
6WIG, pp. 173, 250. 
7WIG, p. 67.
8WIG, pp. 150 ff. 
9WIG, p. 85.
10WIC, pp. 118 ff, 333 ft. 
11WIG, pp. 138-45.
I2WIG, pp. 64-67. 
13WIG, pp. 86-87. 
14WIG, p. 150.
15WIG, pp. 145-46. 
16GWIG, pp. 107-14. 
17WIG, p. 94.
18WIG, p. 151. 
19WIG, p. 58.
20W1G, pp. 55-60. 
21WIG, p. 63.
22W1G, p. 143.
23W1G, throughout part I. '
24W1G, pp. 145-50.
25 WIG. pp. 69-74.
26WIC, pp. 250-51, 264-65. 
27WIG, pp. 244, 302.
28 WIG, 1). 239.
29WIG, pp. 234 ff. 
30WIG, pp. 215 ft. 
31WIC, pp. 333 ff. 52 
32WIG, pp. 251-58. 25 
33WIG, pp. 266 ft.
34WIC, pp. 94, 380, 382.
35According to Patten, the flood and last ice age were caused by a close approach of Mercury; The Biblical Flood and the Ice Epoch (Seattle: Pacific Meridian, 1966), p. 312. 
36 WIG, pp. 25-29
37Fred Hoyle. Astronomy (Garden City, N.Y.: Doubleday, 1962), pp. 269-81; H.P. Berlage, The Origin of the Solar System (Oxford: Pergamon, 1968).
38H.J. Eckelmann and B.C. Newman, slide-illustrated lectures on Genesis chapter one and the origin of the solar system. 39Patten, op. cit., pp. 259 ff.
40WIC, pp. 374, 384.
41George Ahell, Exploration of the Universe (New York: Holt, Rinehart and Winston, 1964), p. 623.
42Samuel Selby, ed., CRC Standard Mathematical Tables, 19th ed. (Cleveland: Chemical Rubber Co., 1971).
43Lloyd Motz and Anneta Duveen. Essentials of Astronomy (Belmont, Calif.: Wadsworth, 1966), p. 227.
44Ibid., p. 230. 
45Abcll, op. cit., pp. 289-90.
46W.E. Forsythe, ed., Smithsonian Physical Tables, 9th ed. (Washington, D.C.: Smithsonian Institution, 1969), p. 729.
47Ibid., p. 739. 
48lbid., p. 780. 
49Motz and Duveen, op. cit., p. 108.
50WIG, IC. 386.
51F.W, Sears and MW. Zemaosky, University Physics, 4th ed. (Reading, Mass.: AddisonWesley, 1970), vol. II, p. 441.
52Forsythe, op. cit., p. 470.
53WIC, p. 386.
54Jack Finegan, Handbook of Biblical Chronology (Princeton, N.J.: Princeton University, 1964), pp. 77-79.
55G.S. Payley and N. Abrahamsen, Science, vol. 179, pp. 892-93 (1973).
56Motz and Duveen, op. cit., p. 235.

I suggest that the near-collision of planets within the span of human history is a dead end as a mechanism for catastrophism.

571 would like to thank my brother James I. Newman for his help in preparing the diagrams for this paper.